Seminar of differential equations will continue on May 15, 2023 at 12pm in lecture room M5.

Roberta Fabbri (Mathematics and Computer Science "Ulisse Dini", University of Florence)

Some questions concerning nonautonomous linear Hamiltonian systems

Abstract:
In the talk the definitions and properties of the rotation number and exponential dichotomy for linear non-autonomous Hamiltonian systems are presented. Some applications to spectral theory for properly perturbed Hamiltonian systems and linear quadratic minimization problems are also presented. Finally, starting from the geometric definition of the rotation number related to the Arnold-Maslov index, some ideas on numerical computation of the Maslov index for frames, i.e., Lagrange planes generated by the solutions of the systems, are given. This is related to the spectral theory of the \(n\)-dimensional Schrödinger equation.

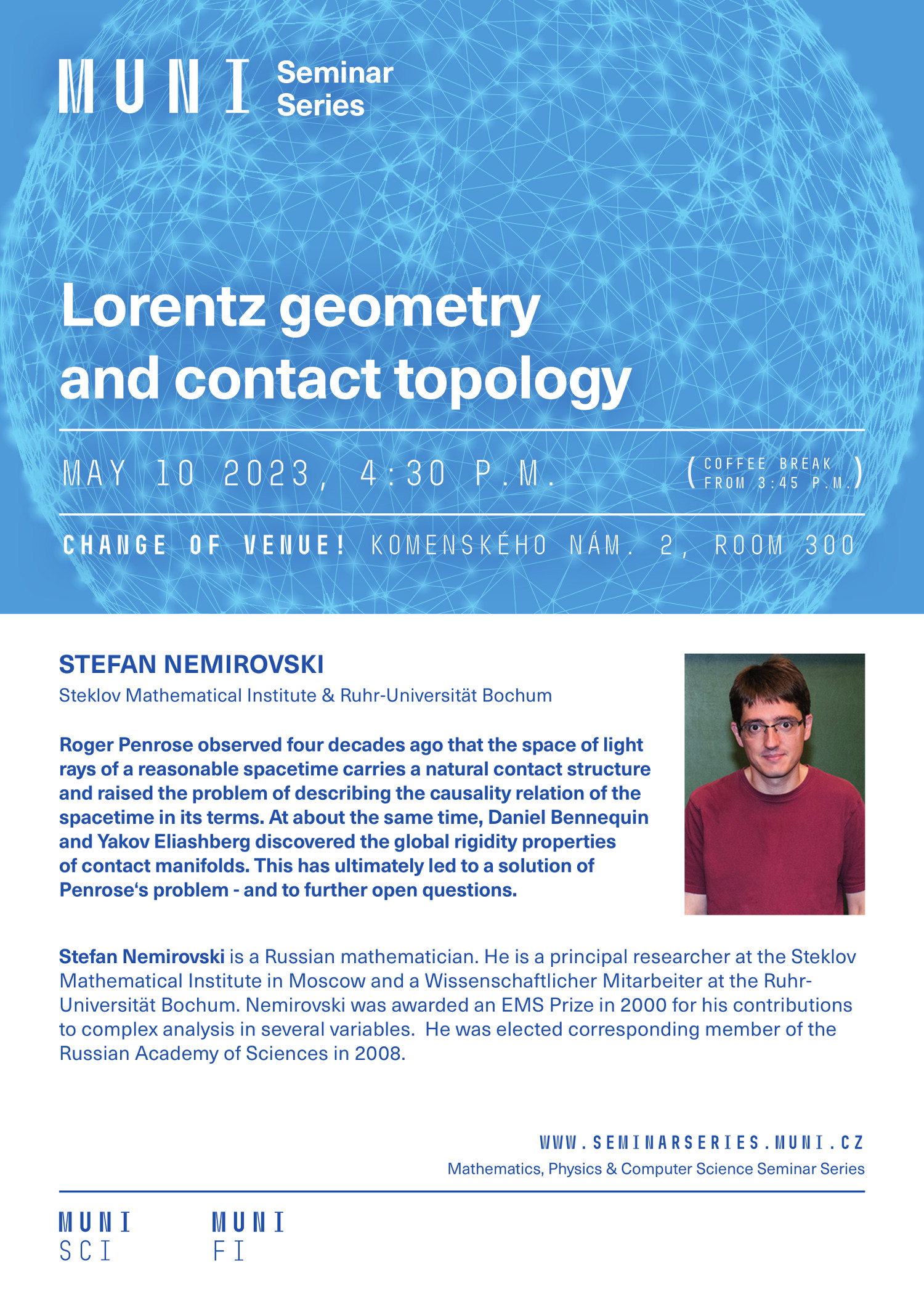
Mathematics, Physics & Computer Science Seminar Series

10 May 2023, 4:30PM, Meeting room nr. 300, Komenského náměstí 220/2

Stefan Nemirovski

Lorentz geometry and contact topology

Stefan Nemirovski is a Russian mathematician. He is a principal researcher at the Steklov Mathematical Institute in Moscow and a Wissenschaftlicher Mitarbeiter at the Ruhr-Universität Bochum. Nemirovski was awarded an EMS Prize in 2000 for his contributions to complex analysis in several variables. He was elected corresponding member of the Russian Academy of Sciences in 2008.

Radek Suchanek will defend his thesis "Geometric Structures and Methods in View of Their Applications in Mathematical Physics" in the specialization Geometry, Topology and Geometric Analysis of our doctoral programme (chair of the committee - prof. J. Janyška, members - prof. M. Doupovec, prof. V. Roubtsov, prof. J. Slovák, doc. J. Hrdina, referees - I. Roulstone (GB), Giovanni Ortenzi (Italy), Kushner Alexei (Russia).

He studied under two supervisions within a cotutelle agreement, prof. J. Slovák was the supervisor in Brno, prof. Vladimir Roubtsov was the supervisor at the University of Angérs, France.

The defence will take place on 29 March, 2023, 2 pm, in the meeting room of our department. The defence will be also available on ZOOM

The complete archive of the dissertation is HERE.

Innolec lectures dates: 4.4. - 5.4.2023, from 2pm to 4pm, 6.4.2023 from 10am to 11:30am and 1pm to 2:30pm, the lectures will be in the Seminar room on the 1st floor.

Franc Forstnerič, University of Ljubljana

Title: Oka manifolds and applications

Abstract:

I will present some recent developments in the theory of Oka manifolds and their applications. After a brief review of the classical Oka-Grauert theory, I shall recall the notion of an Oka manifold and an Oka map, present the two main characterisations of this class of manifolds and maps, discuss the methods to find new Oka manifolds from known ones, and survey the examples. Time permitting, I shall discuss applications of Oka theory in the classical theory of minimal surfaces in Euclidean spaces.